A) and
B) and
C) and
D) and
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the center-radius form of the equation of the circle. -center , radius
A)
B)
C)
D)
Correct Answer

verified
A
Correct Answer
verified
Multiple Choice
Write an equation for the line described. Give your answer in slope-intercept form. -vertical, through (4, -1)
A) x = -1
B) x = 4
C) y = -1
D) y = 4
Correct Answer

verified
B
Correct Answer
verified
Multiple Choice
Use a graphing calculator to solve the linear equation.
-Rewrite the equation so that one side is 0 , then replace 0 with . The graph of the equation for is shown. Use the graph to determine the solution of the equation.
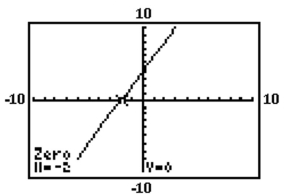
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the slope of the line satisfying the given conditions. -through and
A) undefined
B) 5
C)
D) 0
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Solve the problem. Write all linear equations in slope-intercept form. -Suppose that a sales person observes that if an item is priced at per item then 7 items are sold. If 5 items are sold for per item then find a linear equation to model the number of items sold for dollars per item. Find the slope-intercept form of the equation of the line.
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Decide whether the relation defines a function.
-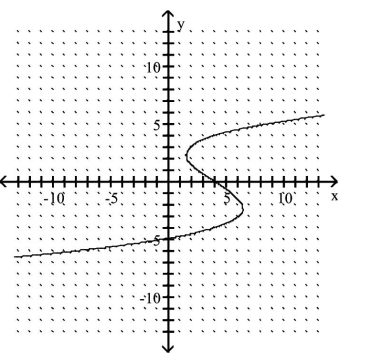
A) Function
B) Not a function
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Write an equation for the line described. Write the equation in the form specified. -parallel to , through ; slope-intercept form
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
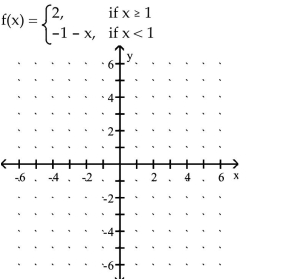
Graph the function.
-
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Match the equation with the correct graph. -
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Graph the function.
-
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine whether the three points are the vertices of a right triangle. -(9, 1) , (15, 3) , (14, -2)
A) Yes
B) No
Correct Answer

verified
B
Correct Answer
verified
Multiple Choice
Give a rule for the piecewise-defined function. Then give the domain and range.
-![Give a rule for the piecewise-defined function. Then give the domain and range. - A) f ( x ) = \left\{ \begin{array} { l l } 4 & \text { if } x < 0 \\ - 4 x & \text { if } x \geq 0 \end{array} ; \right. Domain: ( - \infty , 0 ) \cup \{ 4 \} , Range: ( - \infty , \infty ) B) f ( x ) = \left\{ \begin{array} { l } 4 \text { if } x < 0 \\ x \text { if } x \geq 0 \end{array} ; \right. Domain: ( - \infty , 0 ] \cup ( 4 ) , Range: ( - \infty , \infty ) C) f ( x ) = \left\{ \begin{array} { l l } 4 & \text { if } x \leq 0 \\ - x & \text { if } x > 0 \end{array} ; \right. Domain: ( - \infty , \infty ) , Range: ( - \infty , 0 ) \cup \{ 4 \} D) f ( x ) = \left\{ \begin{array} { l l } 4 & \text { if } x < 0 \\ - x & \text { if } x \geq 0 \end{array} ; \right. Domain: ( - \infty , \infty ) , Range: ( - \infty , 0 ] \cup \{ 4 \}](https://d2lvgg3v3hfg70.cloudfront.net/TB4654/11ecc6b8_4c50_5d96_a77c_d14bbc255471_TB4654_11.jpg)
A) Domain: , Range:
B) Domain: , Range:
C) Domain: , Range:
D) Domain: , Range:
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Evaluate the function. -Find when
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine if the function is even, odd, or neither. -
A) Neither
B) Even
C) Odd
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Graph the line and give the domain and the range. -
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine whether the three points are the vertices of a right triangle. -(-5, 11) , (-3, 15) , (-1, 14)
A) Yes
B) No
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the average rate of change illustrated in the graph.
-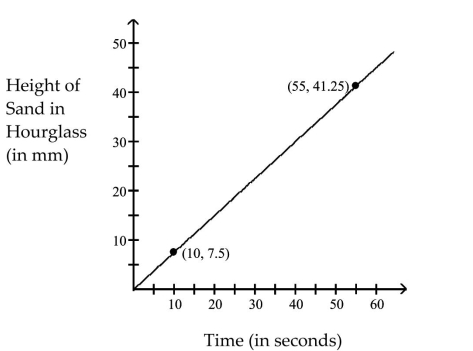
A) per second
B) per second
C) per second
D) per second
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Give the domain and range of the relation. -
A) None of these
B) domain: ; range:
C) domain: ; range:
D) domain: ; range:
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Graph the linear function and give the domain and the range. If the function is a constant function, identify it as such.
-
A) ![]()
B) ![]()
C) D = ( - \infty , \infty ) , R = ( - \infty , \infty ) \) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Showing 1 - 20 of 525
Related Exams