Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Probabilities Involving Permutations and Combinations -Amy, Jean, Keith, Tom, Susan, and Dave have all been invited to a birthday party. They arrive randomly and each person arrives at a different time. In how many ways can they arrive? In how many ways can Jean arrive first and Keith last? Find the probability that Jean will arrive first and Keith will arrive last.
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute and Interpret Probabilities Using the Classical Method -A single die is rolled twice. The set of 36 equally likely outcomes is , , , . Find the probability of getting two numbers whose sum is greater than 10 .
A)
B)
C)
D) 3
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute At-least Probabilities
-Two companies, A and B, package and market a chemical substance and claim of the total weight of the substance is sodium. However, a careful survey of 4,000 packages (half from each company) indicates that the proportion varies around , with the results shown below. Find the percentage of all chemical B packages that contain a sodium total weight proportion above .

A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Solve Counting Problems Using the Multiplication Rule -
A) 700
B) 489,300
C) 1
D) 699
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine the Appropriate Counting Technique to Use -How many different arrangements are possible using 6 letters from the word PAYMENT
A) 5040
B) 2520
C) 7
D) 42
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Solve Counting Problems Using the Multiplication Rule -A medical salesperson is to visit the various members of the staff at a clinic. He must see 8 doctors, 6 physicians assistants, 12 nurses, 3 medical technologists, and 3 receptionists. How many different ways can these people be visited by the salesperson if the order is not important
A) 5184
B) 32
C) 25,920
D) 6,220,800
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Solve Counting Problems Using the Multiplication Rule -How many different breakfasts can you have at the local diner if you can select 3 different egg dishes (scrambled, fried, poached) , 4 choices of meat (steak, ham, bacon, sausage) , 5 breads (white, wheat, rye, muffin, bagel) , 6 juices (tomato, grape, apple, orange, mixed berry, vegetable cocktail) , and 4 beverages (water, milk, coffee, tea)
A) 1440
B) 5
C) 22
D) 360
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Probabilities Using the General Multiplication Rule -Given events A and B with probabilities P(A) = 0.75 and P(B) = 0.15, are A and B mutually exclusive
A) cannot be determined
B) no
C) yes
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Probabilities Involving Permutations and Combinations -If you are dealt 6 cards from a shuffled deck of 52 cards, find the probability of getting 3 jacks and 3 aces.
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Probabilities Using the General Multiplication Rule -Numbered disks are placed in a box and one disk is selected at random. If there are 6 red disks numbered 1 through 6, and 8 yellow disks numbered 7 through 14 , find the probability of selecting a red disk, given that an odd-numbered disk is selected.
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Conditional Probabilities
-The managers of a corporation were surveyed to determine the background that leads to a successful manager. Each manager was rated as being either a good, fair, or poor manager by his/her boss. The manager's educational background was also noted. The data appear below. Given that a manager is a good manager, what is the probability that this manager has some college background?
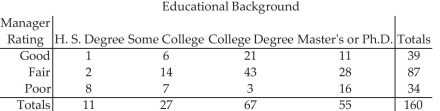
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Probabilities Involving Permutations and Combinations -To play the lottery in a certain state, a person has to correctly select 5 out of 45 numbers, paying for each five-number selection. If the five numbers picked are the same as the ones drawn by the lottery, an enormous sum of money is bestowed. What is the probability that a person with one combination of five numbers will win? What is the probability of winning if 100 different lottery tickets are purchased?
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute the Probability of an Event Using the Complement Rule -You are dealt one card from a 52 -card deck. Find the probability that you are not dealt a
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine the Appropriate Counting Technique to Use -License plates are made using 3 letters followed by 2 digits. How many plates can be made if repetition of letters and digits is allowed
A) 1,757,600
B) 100,000
C) 11,881,376
D) 175,760
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Solve Counting Problems Using the Multiplication Rule -You are taking a multiple-choice test that has 11 questions. Each of the questions has 5 choices, with one correct choice per question. If you select one of these options per question and leave nothing blank, in how many ways can you answer the questions
A) 48,828,125
B) 55
C) 161,051
D) 16
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute and Interpret Probabilities Using the Classical Method
-Use the spinner below to answer the question. Assume that it is equally probable that the pointer will land on any one of the five numbered spaces. If the pointer lands on a borderline, spin again.
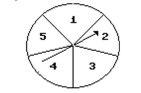 Find the probability that the arrow will land on an odd number.
Find the probability that the arrow will land on an odd number.
A)
B)
C) 1
D) 0
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Probabilities Using the General Multiplication Rule -Numbered disks are placed in a box and one disk is selected at random. If there are 3 red disks numbered 1 through 3 , and 2 yellow disks numbered 4 through 5 , find the probability of selecting a disk numbered 3 , given that a red disk is selected.
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Solve Counting Problems Involving Permutations with Nondistinct Items -How many distinct arrangements of the letters in the word football are possible
A) 10,080
B) 1680
C) 720
D) 40,320
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Compute Probabilities Using the General Multiplication Rule -Assume that P(E) = 0.15 and P(F) = 0.48. If E and F are independent, find P(E and F) .
A) 0.072
B) 0.15
C) 0.558
D) 0.630
Correct Answer

verified
Correct Answer
verified
Showing 241 - 260 of 266
Related Exams