A) −28.38%.
B) −41.72%.
C) −69.49%.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions lena liecken is a senior bond analyst at taurus investment Management. Kristel Kreming, a junior analyst, works for liecken in helping conduct fixed-income research for the firm's portfolio managers. liecken and Kreming meet to discuss several bond positions held in the firm's portfolios. bonds i and ii both have a maturity of one year, an annual coupon rate of 5%, and a mar- ket price equal to par value. The risk-free rate is 3%. historical default experiences of bonds comparable to bonds i and ii are presented in exhibit 1. EXHIBIT 1 Credit Risk Information for Comparable Bonds Bond III Bond III is a zero-coupon bond with three years to maturity. Liecken evaluates similar bonds and estimates a recovery rate of and a risk-neutral default probability of , assuming conditional probabilities of default. Kreming creates Exhibit 2 to compute Bond III's credit valuation adjustment. She assumes a flat yield curve at , with exposure, recovery, and loss given default values expressed per 100 of par value. Bond IV Bond IV is an AA rated bond that matures in five years, has a coupon rate of , and a modified duration of 4.2. Liecken is concerned about whether this bond will be downgraded to an A rating, but she does not expect the bond to default during the next year. Kreming constructs a partial transition matrix, which is presented in Exhibit 3, and suggests using a model to predict the rating change of Bond IV using leverage ratios, return on assets, and macroeconomic variables. Default Probabilities Kreming calculates the risk-neutral probabilities, compares them with the actual default probabilities of bonds evaluated over the past 10 years, and observes that the actual and risk-neutral probabilities differ. She makes two observations regarding the comparison of these probabilities: Observation 1: Actual default probabilities include the default risk premium associated with the uncertainty in the timing of the possible default loss. Observation 2: The observed spread over the yield on a risk-free bond in practice includes liquidity and tax considerations, in addition to credit risk. -based on exhibit 3, if bond iV's credit rating changes during the next year to an a rating, its expected price change would be closest to:
A) −8.00%.
B) −7.35%.
C) −3.15%.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions lena liecken is a senior bond analyst at taurus investment Management. Kristel Kreming, a junior analyst, works for liecken in helping conduct fixed-income research for the firm's portfolio managers. liecken and Kreming meet to discuss several bond positions held in the firm's portfolios. bonds i and ii both have a maturity of one year, an annual coupon rate of 5%, and a mar- ket price equal to par value. The risk-free rate is 3%. historical default experiences of bonds comparable to bonds i and ii are presented in exhibit 1. EXHIBIT 1 Credit Risk Information for Comparable Bonds Bond III Bond III is a zero-coupon bond with three years to maturity. Liecken evaluates similar bonds and estimates a recovery rate of and a risk-neutral default probability of , assuming conditional probabilities of default. Kreming creates Exhibit 2 to compute Bond III's credit valuation adjustment. She assumes a flat yield curve at , with exposure, recovery, and loss given default values expressed per 100 of par value. Bond IV Bond IV is an AA rated bond that matures in five years, has a coupon rate of , and a modified duration of 4.2. Liecken is concerned about whether this bond will be downgraded to an A rating, but she does not expect the bond to default during the next year. Kreming constructs a partial transition matrix, which is presented in Exhibit 3, and suggests using a model to predict the rating change of Bond IV using leverage ratios, return on assets, and macroeconomic variables. Default Probabilities Kreming calculates the risk-neutral probabilities, compares them with the actual default probabilities of bonds evaluated over the past 10 years, and observes that the actual and risk-neutral probabilities differ. She makes two observations regarding the comparison of these probabilities: Observation 1: Actual default probabilities include the default risk premium associated with the uncertainty in the timing of the possible default loss. Observation 2: The observed spread over the yield on a risk-free bond in practice includes liquidity and tax considerations, in addition to credit risk. -The expected exposure to default loss for bond i is:
A) less than the expected exposure for bond ii.
B) the same as the expected exposure for bond ii.
C) greater than the expected exposure for bond ii.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions
daniela ibarra is a senior analyst in the fixed-income department of a large wealth manage-ment firm. Marten Koning is a junior analyst in the same department, and david lok is a member of the credit research team.
The firm invests in a variety of bonds. ibarra is presently analyzing a set of bonds with some similar characteristics, such as four years until maturity and a par value of €1,000. exhibit 1 includes details of these bonds.
exhibit 1 a brief description of the bonds being analyzed bond descriptionb1 a zero-coupon, four-year corporate bond with a par value of €1,000. The wealth management firm's research team has estimated that the risk-neutral probability of default (the hazard rate) for each date for the bond is 1.50%, and the recovery rate is 30%.b2 a bond similar to b1, except that it has a fixed annual coupon rate of 6% paid annually.
b3 a bond similar to b2 but rated aa.
b4 a bond similar to b2 but the coupon rate is the one-year benchmark rate plus 4%.ibarra asks Koning to assist her with analyzing the bonds. She wants him to perform the analysis with the assumptions that there is no interest rate volatility and that the government bond yield curve is flat at 3%.ibarra performs the analysis assuming an upward-sloping yield curve and volatile interest rates. exhibit 2 provides the data on annual payment benchmark government bonds.1 She uses these data to construct a binomial interest rate tree (shown in exhibit 3) based on an assump-tion of future interest rate volatility of 20%.
1 For simplicity, this exhibit uses
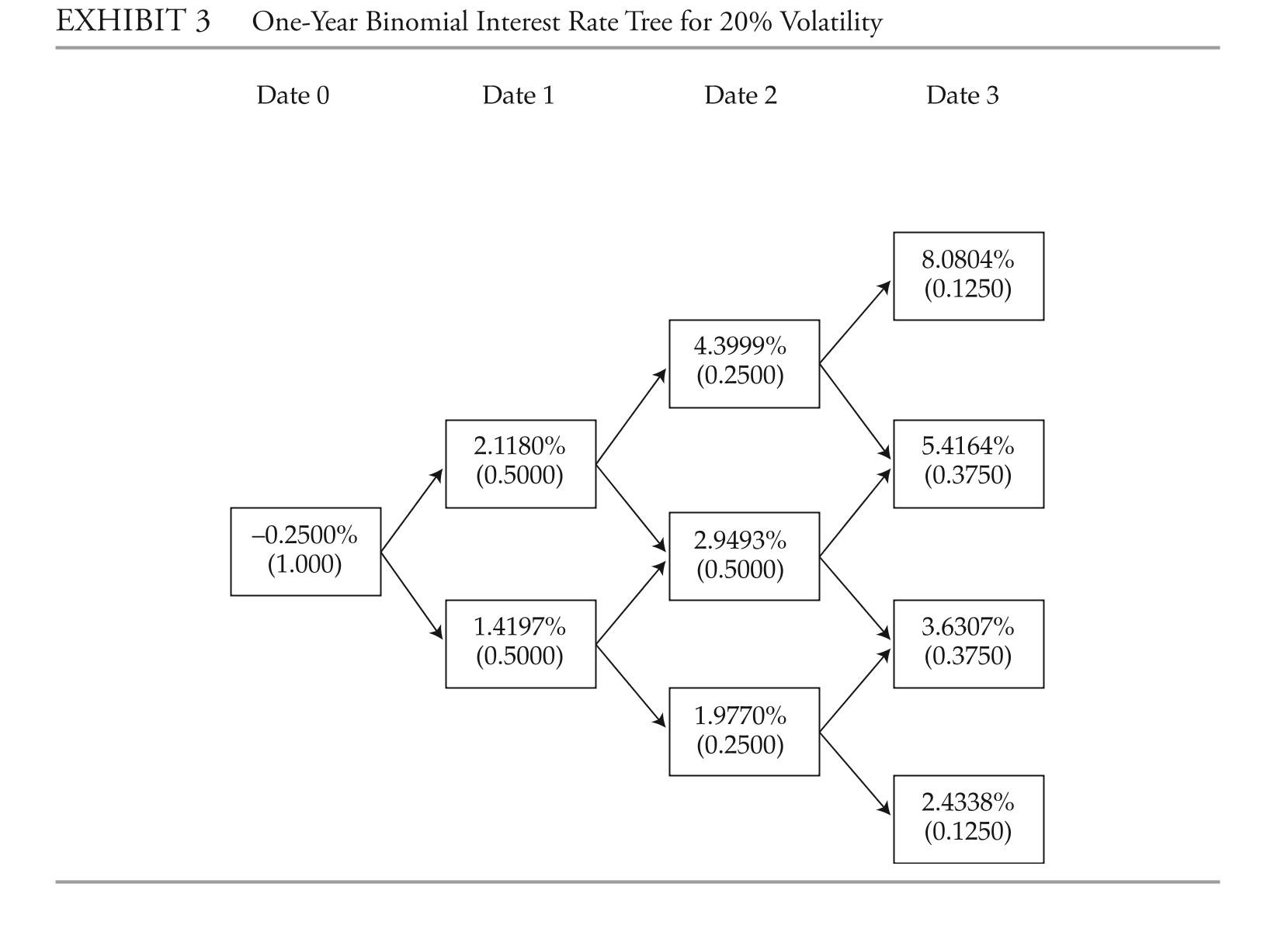 answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-Floating-rate note b4 is currently rated bbb by Standard & Poor's and Fitch ratings (and baa by Moody's investors Service) . based on the research department assumption about
The probability of default in Question 10 and her own assumption in Question 11, which
Action does ibarra most likely expect from the credit rating agencies?
answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-Floating-rate note b4 is currently rated bbb by Standard & Poor's and Fitch ratings (and baa by Moody's investors Service) . based on the research department assumption about
The probability of default in Question 10 and her own assumption in Question 11, which
Action does ibarra most likely expect from the credit rating agencies?
A) downgrade from bbb to bb
B) Upgrade from bbb to aaa
C) Place the issuer on watch with a positive outlook
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions
daniela ibarra is a senior analyst in the fixed-income department of a large wealth manage-ment firm. Marten Koning is a junior analyst in the same department, and david lok is a member of the credit research team.
The firm invests in a variety of bonds. ibarra is presently analyzing a set of bonds with some similar characteristics, such as four years until maturity and a par value of €1,000. exhibit 1 includes details of these bonds.
exhibit 1 a brief description of the bonds being analyzed bond descriptionb1 a zero-coupon, four-year corporate bond with a par value of €1,000. The wealth management firm's research team has estimated that the risk-neutral probability of default (the hazard rate) for each date for the bond is 1.50%, and the recovery rate is 30%.b2 a bond similar to b1, except that it has a fixed annual coupon rate of 6% paid annually.
b3 a bond similar to b2 but rated aa.
b4 a bond similar to b2 but the coupon rate is the one-year benchmark rate plus 4%.ibarra asks Koning to assist her with analyzing the bonds. She wants him to perform the analysis with the assumptions that there is no interest rate volatility and that the government bond yield curve is flat at 3%.ibarra performs the analysis assuming an upward-sloping yield curve and volatile interest rates. exhibit 2 provides the data on annual payment benchmark government bonds.1 She uses these data to construct a binomial interest rate tree (shown in exhibit 3) based on an assump-tion of future interest rate volatility of 20%.
1 For simplicity, this exhibit uses
 answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-during the presentation about how the research team estimates the probability of default for a particular bond issuer, lok is asked for his thoughts on the shape of the
Term structure of credit spreads. Which statement is he most likely to include in his
Response?
answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-during the presentation about how the research team estimates the probability of default for a particular bond issuer, lok is asked for his thoughts on the shape of the
Term structure of credit spreads. Which statement is he most likely to include in his
Response?
A) The term structure of credit spreads typically is flat or slightly upward sloping for high-quality investment-grade bonds. high-yield bonds are more sensitive to the
Credit cycle, however, and can have a more upwardly sloped term structure of credit
Spreads than investment-grade bonds or even an inverted curve.
B) The term structure of credit spreads for corporate bonds is always upward sloping, the more so the weaker the credit quality because probabilities of default are positively
Correlated with the time to maturity.
C) There is no consistent pattern to the term structure of credit spreads. The shape of the credit term structure depends entirely on industry factors.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions
anna lebedeva is a fixed-income portfolio manager. Paulina Kowalski, a junior analyst, and lebedeva meet to review several positions in lebedeva's portfolio.
lebedeva begins the meeting by discussing credit rating migration. Kowalski asks leb-edeva about the typical impact of credit rating migration on the expected return on a bond.
lebedeva asks Kowalski to estimate the expected return over the next year on a bond issued byentre corp. The bbb rated bond has a yield to maturity of 5.50% and a modified duration of 7.54. Kowalski calculates the expected return on the bond over the next year given the partial
credit transition and credit spread data in exhibit 1. She assumes that market spreads and yields will remain stable over the year. lebedeva next asks Kowalski to analyze a three-year bond, issued by Vrairive S.a., using an arbitrage-free framework. The bond's coupon rate is 5%, with interest paid annually and a par value of 100. in her analysis, she makes the following three assumptions:
• The annual interest rate volatility is 10%.
• The recovery rate is one-third of the exposure each period.
• The hazard rate, or conditional probability of default each year, is 2.00%.
Selected information on benchmark government bonds for the Vrairive bond is presented
in exhibit 2, and the relevant binomial interest rate tree is presented in exhibit 3. 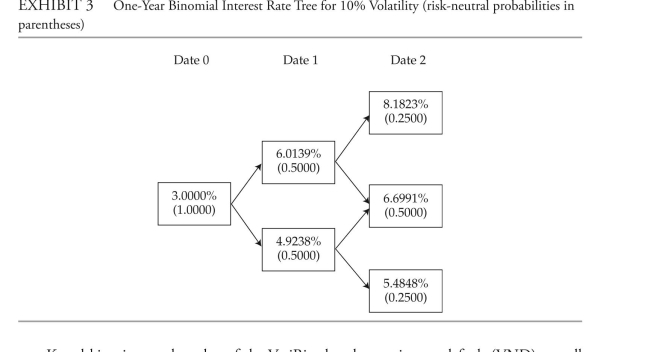 Kowalski estimates the value of the Vrairive bond assuming no default (Vnd) as wellas the fair value of the bond. She then estimates the bond's yield to maturity and the bond'scredit spread over the benchmark in exhibit 2. Kowalski asks lebedeva, "What might cause the bond's credit spread to decrease?"
lebedeva and Kowalski next discuss the drivers of the term structure of credit spreads.Kowalski tells lebedeva:Statement 1: The credit term structure for the most highly rated securities tends to be either flat or slightly upward sloping.Statement 2: The credit term structure for lower-rated securities is often steeper, and creditspreads widen with expectations of strong economic growth.next, Kowalski analyzes the outstanding bonds of dll corporation, a high-quality issuerwith a strong, competitive position. her focus is to determine the rationale for a positively sloped credit spread term structure.lebedeva ends the meeting by asking Kowalski to recommend a credit analysis approach
for a securitized asset-backed security (abS) held in the portfolio. This non-static asset pool is made up of many medium-term auto loans that are homogeneous, and each loan is small relative to the total value of the pool.
-Which of Kowalski's statements regarding the term structure of credit spreads is correct?
Kowalski estimates the value of the Vrairive bond assuming no default (Vnd) as wellas the fair value of the bond. She then estimates the bond's yield to maturity and the bond'scredit spread over the benchmark in exhibit 2. Kowalski asks lebedeva, "What might cause the bond's credit spread to decrease?"
lebedeva and Kowalski next discuss the drivers of the term structure of credit spreads.Kowalski tells lebedeva:Statement 1: The credit term structure for the most highly rated securities tends to be either flat or slightly upward sloping.Statement 2: The credit term structure for lower-rated securities is often steeper, and creditspreads widen with expectations of strong economic growth.next, Kowalski analyzes the outstanding bonds of dll corporation, a high-quality issuerwith a strong, competitive position. her focus is to determine the rationale for a positively sloped credit spread term structure.lebedeva ends the meeting by asking Kowalski to recommend a credit analysis approach
for a securitized asset-backed security (abS) held in the portfolio. This non-static asset pool is made up of many medium-term auto loans that are homogeneous, and each loan is small relative to the total value of the pool.
-Which of Kowalski's statements regarding the term structure of credit spreads is correct?
A) only Statement 1
B) only Statement 2
C) both Statement 1 and Statement 2
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions
daniela ibarra is a senior analyst in the fixed-income department of a large wealth manage-ment firm. Marten Koning is a junior analyst in the same department, and david lok is a member of the credit research team.
The firm invests in a variety of bonds. ibarra is presently analyzing a set of bonds with some similar characteristics, such as four years until maturity and a par value of €1,000. exhibit 1 includes details of these bonds.
exhibit 1 a brief description of the bonds being analyzed bond descriptionb1 a zero-coupon, four-year corporate bond with a par value of €1,000. The wealth management firm's research team has estimated that the risk-neutral probability of default (the hazard rate) for each date for the bond is 1.50%, and the recovery rate is 30%.b2 a bond similar to b1, except that it has a fixed annual coupon rate of 6% paid annually.
b3 a bond similar to b2 but rated aa.
b4 a bond similar to b2 but the coupon rate is the one-year benchmark rate plus 4%.ibarra asks Koning to assist her with analyzing the bonds. She wants him to perform the analysis with the assumptions that there is no interest rate volatility and that the government bond yield curve is flat at 3%.ibarra performs the analysis assuming an upward-sloping yield curve and volatile interest rates. exhibit 2 provides the data on annual payment benchmark government bonds.1 She uses these data to construct a binomial interest rate tree (shown in exhibit 3) based on an assump-tion of future interest rate volatility of 20%.
1 For simplicity, this exhibit uses
 answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-ibarra is interested in analyzing how a simultaneous decrease in the recovery rate and the probability of default would affect the fair value of bond b2. She decreases both the
Recovery rate and the probability of default by 25% of their existing estimates and recom-
Putes the bond's fair value. The recomputed fair value is closest to:
answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-ibarra is interested in analyzing how a simultaneous decrease in the recovery rate and the probability of default would affect the fair value of bond b2. She decreases both the
Recovery rate and the probability of default by 25% of their existing estimates and recom-
Putes the bond's fair value. The recomputed fair value is closest to:
A) €1,096.59.
B) €1,108.40.
C) €1,111.91.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions
anna lebedeva is a fixed-income portfolio manager. Paulina Kowalski, a junior analyst, and lebedeva meet to review several positions in lebedeva's portfolio.
lebedeva begins the meeting by discussing credit rating migration. Kowalski asks leb-edeva about the typical impact of credit rating migration on the expected return on a bond.
lebedeva asks Kowalski to estimate the expected return over the next year on a bond issued byentre corp. The bbb rated bond has a yield to maturity of 5.50% and a modified duration of 7.54. Kowalski calculates the expected return on the bond over the next year given the partial
credit transition and credit spread data in exhibit 1. She assumes that market spreads and yields will remain stable over the year. lebedeva next asks Kowalski to analyze a three-year bond, issued by Vrairive S.a., using an arbitrage-free framework. The bond's coupon rate is 5%, with interest paid annually and a par value of 100. in her analysis, she makes the following three assumptions:
• The annual interest rate volatility is 10%.
• The recovery rate is one-third of the exposure each period.
• The hazard rate, or conditional probability of default each year, is 2.00%.
Selected information on benchmark government bonds for the Vrairive bond is presented
in exhibit 2, and the relevant binomial interest rate tree is presented in exhibit 3.  Kowalski estimates the value of the Vrairive bond assuming no default (Vnd) as wellas the fair value of the bond. She then estimates the bond's yield to maturity and the bond'scredit spread over the benchmark in exhibit 2. Kowalski asks lebedeva, "What might cause the bond's credit spread to decrease?"
lebedeva and Kowalski next discuss the drivers of the term structure of credit spreads.Kowalski tells lebedeva:Statement 1: The credit term structure for the most highly rated securities tends to be either flat or slightly upward sloping.Statement 2: The credit term structure for lower-rated securities is often steeper, and creditspreads widen with expectations of strong economic growth.next, Kowalski analyzes the outstanding bonds of dll corporation, a high-quality issuerwith a strong, competitive position. her focus is to determine the rationale for a positively sloped credit spread term structure.lebedeva ends the meeting by asking Kowalski to recommend a credit analysis approach
for a securitized asset-backed security (abS) held in the portfolio. This non-static asset pool is made up of many medium-term auto loans that are homogeneous, and each loan is small relative to the total value of the pool.
-based on exhibit 1, the one-year expected return on the entre corp. bond is closest to:
Kowalski estimates the value of the Vrairive bond assuming no default (Vnd) as wellas the fair value of the bond. She then estimates the bond's yield to maturity and the bond'scredit spread over the benchmark in exhibit 2. Kowalski asks lebedeva, "What might cause the bond's credit spread to decrease?"
lebedeva and Kowalski next discuss the drivers of the term structure of credit spreads.Kowalski tells lebedeva:Statement 1: The credit term structure for the most highly rated securities tends to be either flat or slightly upward sloping.Statement 2: The credit term structure for lower-rated securities is often steeper, and creditspreads widen with expectations of strong economic growth.next, Kowalski analyzes the outstanding bonds of dll corporation, a high-quality issuerwith a strong, competitive position. her focus is to determine the rationale for a positively sloped credit spread term structure.lebedeva ends the meeting by asking Kowalski to recommend a credit analysis approach
for a securitized asset-backed security (abS) held in the portfolio. This non-static asset pool is made up of many medium-term auto loans that are homogeneous, and each loan is small relative to the total value of the pool.
-based on exhibit 1, the one-year expected return on the entre corp. bond is closest to:
A) 3.73%.
B) 5.50%.
C) 7.27%.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions
daniela ibarra is a senior analyst in the fixed-income department of a large wealth manage-ment firm. Marten Koning is a junior analyst in the same department, and david lok is a member of the credit research team.
The firm invests in a variety of bonds. ibarra is presently analyzing a set of bonds with some similar characteristics, such as four years until maturity and a par value of €1,000. exhibit 1 includes details of these bonds.
exhibit 1 a brief description of the bonds being analyzed bond descriptionb1 a zero-coupon, four-year corporate bond with a par value of €1,000. The wealth management firm's research team has estimated that the risk-neutral probability of default (the hazard rate) for each date for the bond is 1.50%, and the recovery rate is 30%.b2 a bond similar to b1, except that it has a fixed annual coupon rate of 6% paid annually.
b3 a bond similar to b2 but rated aa.
b4 a bond similar to b2 but the coupon rate is the one-year benchmark rate plus 4%.ibarra asks Koning to assist her with analyzing the bonds. She wants him to perform the analysis with the assumptions that there is no interest rate volatility and that the government bond yield curve is flat at 3%.ibarra performs the analysis assuming an upward-sloping yield curve and volatile interest rates. exhibit 2 provides the data on annual payment benchmark government bonds.1 She uses these data to construct a binomial interest rate tree (shown in exhibit 3) based on an assump-tion of future interest rate volatility of 20%.
1 For simplicity, this exhibit uses
 answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-Koning realizes that an increase in the recovery rate would lead to an increase in the bond's fair value, whereas an increase in the probability of default would lead to a decrease in the
Bond's fair value. he is not sure which effect would be greater, however. So, he increases
Both the recovery rate and the probability of default by 25% of their existing estimates
And recomputes the bond's fair value. The recomputed fair value is closest to:
answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-Koning realizes that an increase in the recovery rate would lead to an increase in the bond's fair value, whereas an increase in the probability of default would lead to a decrease in the
Bond's fair value. he is not sure which effect would be greater, however. So, he increases
Both the recovery rate and the probability of default by 25% of their existing estimates
And recomputes the bond's fair value. The recomputed fair value is closest to:
A) €843.14.
B) €848.00.
C) €855.91.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
The following information relates to Questions
daniela ibarra is a senior analyst in the fixed-income department of a large wealth manage-ment firm. Marten Koning is a junior analyst in the same department, and david lok is a member of the credit research team.
The firm invests in a variety of bonds. ibarra is presently analyzing a set of bonds with some similar characteristics, such as four years until maturity and a par value of €1,000. exhibit 1 includes details of these bonds.
exhibit 1 a brief description of the bonds being analyzed bond descriptionb1 a zero-coupon, four-year corporate bond with a par value of €1,000. The wealth management firm's research team has estimated that the risk-neutral probability of default (the hazard rate) for each date for the bond is 1.50%, and the recovery rate is 30%.b2 a bond similar to b1, except that it has a fixed annual coupon rate of 6% paid annually.
b3 a bond similar to b2 but rated aa.
b4 a bond similar to b2 but the coupon rate is the one-year benchmark rate plus 4%.ibarra asks Koning to assist her with analyzing the bonds. She wants him to perform the analysis with the assumptions that there is no interest rate volatility and that the government bond yield curve is flat at 3%.ibarra performs the analysis assuming an upward-sloping yield curve and volatile interest rates. exhibit 2 provides the data on annual payment benchmark government bonds.1 She uses these data to construct a binomial interest rate tree (shown in exhibit 3) based on an assump-tion of future interest rate volatility of 20%.
1 For simplicity, this exhibit uses
 answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-as previously mentioned, ibarra is considering a future interest rate volatility of 20% and an upward-sloping yield curve, as shown in exhibit 2. based on her analysis, the fair value
Of bond b2 is closest to:
answer the first five questions (1-4) based on the assumptions made by Marten Koning,the junior analyst. answer questions (8-12) based on the assumptions made by daniela ibarra, the senior analyst.
Note: all calculations in this problem set are carried out on spreadsheets to preserve reci-sion. The rounded results are reported in the solutions.
-as previously mentioned, ibarra is considering a future interest rate volatility of 20% and an upward-sloping yield curve, as shown in exhibit 2. based on her analysis, the fair value
Of bond b2 is closest to:
A) €1,101.24.
B) €1,141.76.
C) €1,144.63.
Correct Answer

verified
Correct Answer
verified
Showing 21 - 30 of 30
Related Exams