Correct Answer

verified
Correct Answer
verified
Short Answer
The region bounded by the given curves is rotated about the specified axis. Find the volume of the resulting solid by any method. about the -axis
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use the method of cylindrical shells to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated axis. Sketch the region and a representative rectangle. the -axis
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Short Answer
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations and inequalities about the -axis.
Correct Answer

verified
Correct Answer
verified
Essay
Use the method of cylindrical shells to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated axis. Sketch the region and a representative rectangle. the -axis
Correct Answer

verified
Correct Answer
verified
Essay
Sketch the region bounded by the graphs of the given equations and find the area of their region.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the area of the region bounded by the given curves.
A) 4
B) 1
C)
D)
E) 2
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Sketch the region enclosed by and . Find the area of the region.
A)
B)
C)
D)
E)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use the Midpoint Rule with to estimate the volume obtained by rotating about the region under the -axis the region under the curve. The choices are rounded to the nearest hundredth. Select the correct answer.
A)
B)
C)
D)
E)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
If of work are needed to stretch a spring from to and another are needed to stretch it from to , what is the natural length of the spring? Round the answer to nearest integer. Select the correct answer.
A)
B)
C)
D)
E)
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the area of the region bounded by the given curves.
A) 2197
B)
C)
D)
E) 6
Correct Answer

verified
Correct Answer
verified
Short Answer
Use a computer algebra system to find the exact volume of the solid obtained by rotating the region bounded by the given curves about the specified line.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the area of the shaded region.
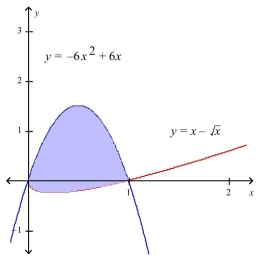
A) 7
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Short Answer
Sketch the region enclosed by the curves , and . Find the area of the region correct to two decimal places.
Correct Answer

verified
Correct Answer
verified
Short Answer
Find the volume of the solid obtained by rotating the region bounded by and about the -axis.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the volume of the solid obtained by rotating the region bounded by and about the -axis. Select the correct answer.
A)
B)
C)
D)
E)
Correct Answer

verified
Correct Answer
verified
Short Answer
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated line. ; the line
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the indicated line. the line
A)
B)
C)
D)
Correct Answer

verified
Correct Answer
verified
Essay
Sketch the region bounded by the graphs of the given equations and find the area of that region.
Correct Answer

verified
Correct Answer
verified
Short Answer
Find the volume of the solid obtained by rotating the region bounded by and about the line .
Correct Answer

verified
Correct Answer
verified
Showing 41 - 60 of 158
Related Exams